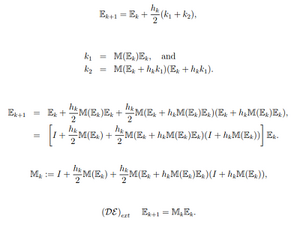G. W. Weber, P. Taylan, B. Akteke-Öztürk, Ö. Uğur, Mathematical and Data Mining Contributions to Dynamics and Optimization of Gene-Environment Networks, Electronic Journal of Theoretical Physics (EJTP), 4, 16(II), pp. 115-146, (December 2007).
G. W. Weber, P. Taylan, B. Akteke-Öztürk, Ö. Uğur, Mathematical and Data Mining Contributions to Dynamics and Optimization of Gene-Environment Networks, in: Crossing in Complexity: Interdisciplinary Application of Physics in Biological and Social Systems, Ignazio Licata and Ammar Sakaji (editors), Nova Publishers, 2010.
ISBN: 978-1-61668-037-4
Abstract
This paper further introduces continuous optimization into the fields of computational biology and environmental protection which belong to the most challenging and emerging areas of science. It refines earlier ones of our models on gene-environment patterns by the use of optimization theory. We emphasize that it bases on and presents work done in [61, 66]. Furthermore, our paper tries to detect and overcome some structural frontiers of our methods applied to the recently introduced gene-environment networks. Based on the experimental data, we investigate the ordinary differential equations having nonlinearities on the right-hand side and a generalized treatment of the absolute shift term which represents the environmental effects. The genetic process is studied by a time-discretization, in particular, Runge-Kutta type discretization. The possibility of detecting stability and instability regions is being shown by a utilization of the combinatorial algorithm of Brayton and Tong which is based on the orbits of polyhedra. The time-continuous and discrete systems can be represented by means of matrices allowing biological implications, they encode and are motivated by our gene-environment networks. A specific contribution of this paper consists in a careful but rigorous integration of the environment into modeling and dynamics, and in further new sights. Relations to parameter estimation within modeling, especially, by using optimization, are indicated, and future research is addressed, especially towards the use of stochastic differential equations. This practically motivated and theoretically elaborated work is devoted for a contribution to better health care, progress in medicine, a better education and more healthy living conditions recommended.
Keywords: computational biology, generalized semi-infinite programming, mathematical modeling, dynamical systems, gene-expression data, environment, stability, structural stability, structural frontiers, continuous, discrete, hybrid, spline, inverse problem, penalization, regularization, stochastic differential equations