Süleyman Cengizci, Ömür Uğur, Srinivasan Natesan, A Stabilized Finite Element Formulation with Shock-Capturing for solving Advection-Dominated Convection–Diffusion Equations having Time-Fractional Derivatives, Journal of Computational Science, 76: 102214 (March 2024, January 2024).
Abstract
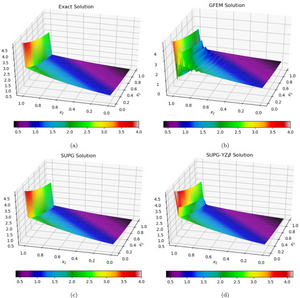
Numerical instabilities arising when simulating transport phenomena dominated by convection processes are among the most challenging situations in computational science, demanding the use of non-classical formulations and techniques to achieve accurate approximations. Although the stabilized formulations usually help suppress node-to-node nonphysical oscillations, numerical approximations typically experience localized instabilities around sharp layers where the solution exhibits rapid changes. We, therefore, propose a stabilized formulation based on the streamline-upwind/Petrov–Galerkin (SUPG) method augmented with YZβ discontinuity-capturing for solving convection-dominated advection-diffusion equations involving Caputo-type time-fractional derivatives numerically. A numerical method based on finite differences is used to approximate the time-fractional derivatives. The nonlinear systems arising from the space and time discretizations are solved with the Newton–Raphson (N–R) method at each time step. The linear equation systems emanating from the N–R processes are then handled with an ILU-preconditioned GMRES search technique. Four test computations are provided to evaluate and compare the performance of the proposed methods and techniques. It is observed that the SUPG-YZβ formulation successfully eliminates nonphysical oscillations.
Keywords: Time-fractional differential equation, Convection-dominated, Finite elements, SUPG-YZβ shock-capturing